Impedance matching is a fundamental aspect of radio frequency (RF) design and testing; Signal reflection caused by impedance mismatch can cause serious problems.

When dealing with theoretical circuits composed of ideal power sources, transmission lines, and loads, matching seems like a trivial operation.

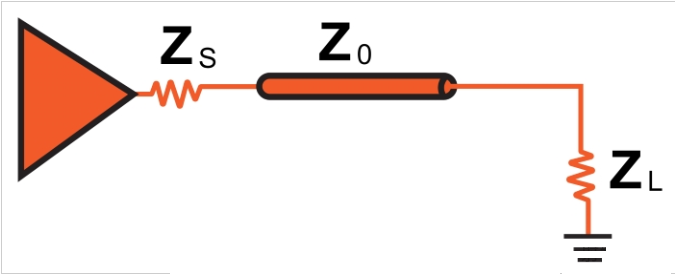
Let's assume that the load impedance is fixed. What we need to do is add a source impedance (ZS) equal to ZL, and then design the transmission line so that its characteristic impedance (Z0) is also equal to ZL.

However, let us temporarily consider the difficulty of implementing this solution in complex RF circuits composed of numerous passive components and integrated circuits. If engineers have to modify each component and specify the size of each microstrip line based on the impedance that serves as the foundation for all other components, the RF design process will become very cumbersome.

In addition, this also assumes that the project has entered the PCB phase. But what if we want to use discrete modules and ready-made cables as interconnects to test and characterize the system? In these cases, compensating for mismatched impedance is even more impractical.

The solution is simple: choose a standardized impedance that can be used for many RF systems and ensure that the design of components and cables matches it. This impedance has been selected; The unit is ohms and the value is 50.

50 ohms

The first thing to understand is that there is nothing particularly special about a 50 Ω impedance itself. This is not a fundamental constant of the universe, although if you frequently deal with RF engineers, you may have this impression. It is not even a fundamental constant in electrical engineering - remember, for example, simply changing the physical dimensions of a coaxial cable will alter its characteristic impedance.

However, a 50 Ω impedance is crucial as most RF systems are designed around this impedance. It is difficult to determine why 50 Ω became the standardized RF impedance, but it is reasonable to speculate that in the early context of coaxial cables, 50 Ω was considered a good compromise choice.

Of course, what is important is not the origin of a specific value, but the benefits of having this standardized impedance. Due to the fact that manufacturers of integrated circuits, fixed attenuators, antennas, etc. take this impedance into consideration when manufacturing components, achieving good matching design is much simpler. In addition, PCB layout has become simpler and clearer, as many engineers share the same goal of designing microstrip and strip lines with a characteristic impedance of 50 Ω.

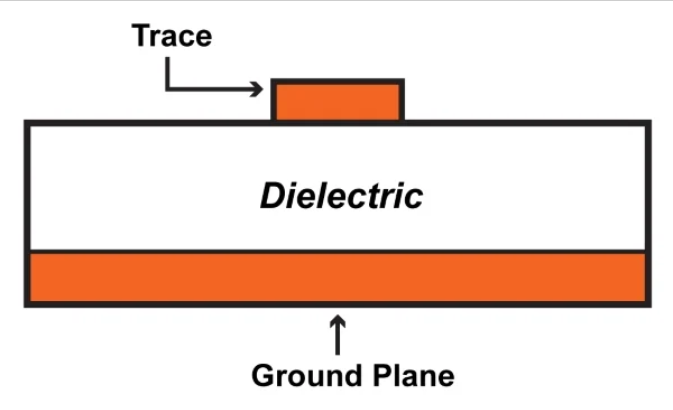
According to the application instructions of Analog Devices, you can create a 50 Ω microstrip line as follows: 1 ounce of copper, a 20 mil wide trace, and a 10 mil gap between the trace and the ground plane (assuming FR-4 dielectric is used).

Before we proceed, it should be clarified that not all high-frequency systems or components are designed for 50 Ω. You can choose other values, but in fact, 75 Ω impedance is still very common. The characteristic impedance of coaxial cable is directly proportional to the natural logarithm of the ratio of its outer diameter (D2) to inner diameter (D1).

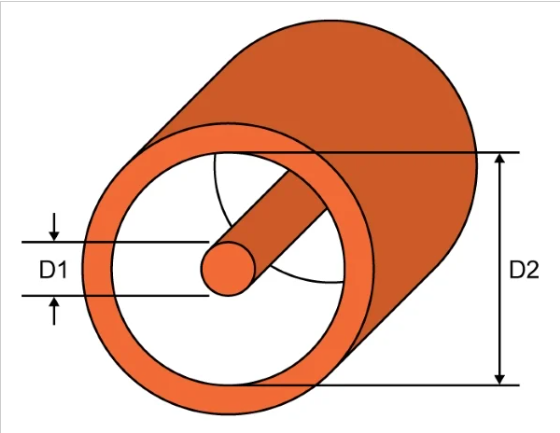
This means that the larger the distance between the inner and outer conductors, the higher the corresponding impedance. A larger gap between two conductors can also result in lower capacitance. Therefore, the capacitance of 75 Ω coaxial cable is lower than that of 50 Ω coaxial cable, which makes 75 Ω cable more suitable for high-frequency digital signals. High frequency digital signals require low capacitance to avoid excessive attenuation of high-frequency components during rapid conversion between logic low and logic high.

reflection coefficient

Considering the importance of impedance matching in RF design, it is not surprising that there is a specific parameter used to represent the quality of matching. This parameter is called the reflection coefficient, with the symbol gamma (Greek capital letter gamma). It is the ratio of the complex amplitude of the reflected wave to the complex amplitude of the incident wave. However, the relationship between the incident wave and the reflected wave is determined by the impedance of the source (ZS) and the load (ZL), so the reflection coefficient can be defined based on these impedances:

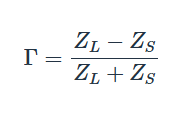
If in this situation, the 'source' is a transmission line, we can change ZS to Z0.

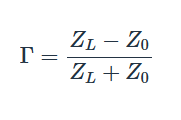
In a typical system, the amplitude of the reflection coefficient is between 0 and 1. Let's take a look at three mathematically intuitive situations to help us understand how reflection coefficients correspond to actual circuit behavior:

If the match is perfect (ZL=Z0), then the molecule is zero, and therefore the reflection coefficient is also zero. This makes sense because a perfect match results in no reflection.

If the load impedance is infinite (i.e. open circuit), the reflection coefficient becomes infinite divided by infinity, and the result is 1. A reflection coefficient of 1 corresponds to complete reflection, meaning that all wave energy is reflected. This makes sense because a transmission line connected to an open circuit corresponds to complete discontinuity (see previous page) - the load cannot absorb any energy, so all energy must be reflected.

If the load impedance is zero (i.e. short circuit), the amplitude of the reflection coefficient becomes Z0 divided by Z0. Therefore, we once again obtain | Gamma |=1, which makes sense because short circuits also correspond to complete discontinuities that cannot absorb any incident wave energy.

Standing Wave Ratio (VSWR)

Another parameter that describes impedance matching is Voltage Standing Wave Ratio (VSWR). Its definition is as follows:

The standing wave ratio (VSWR) approaches impedance matching from the angle of the generated standing wave. It represents the ratio of the highest standing wave amplitude to the lowest standing wave amplitude. This video can help you visualize the relationship between impedance mismatch and standing wave amplitude characteristics, and the following chart shows the standing wave amplitude characteristics for three different reflection coefficients.

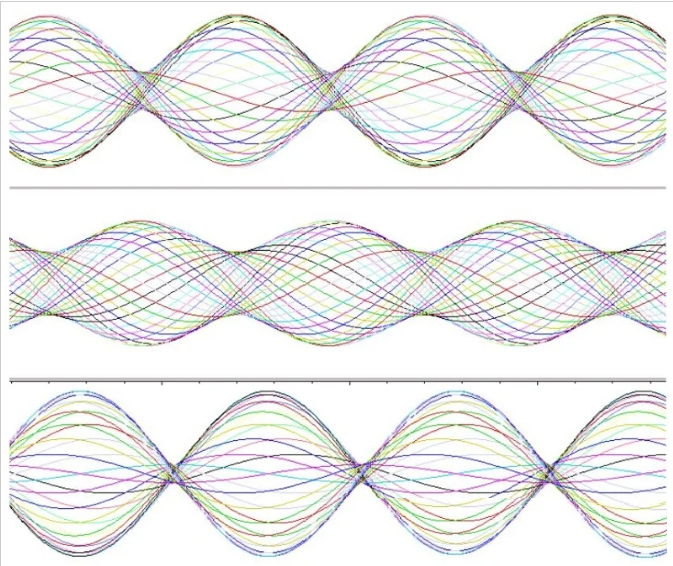
The more severe the impedance mismatch, the greater the difference between the highest and lowest amplitude positions on the standing wave.

The standing wave ratio (VSWR) is usually expressed in the form of a ratio. A perfect match is 1:1, which means that the peak amplitude of the signal remains constant (i.e. there are no standing waves). A ratio of 2:1 indicates that the maximum amplitude of the standing wave caused by reflection is twice its minimum amplitude.

summary

Using standardized impedance makes RF design more practical and efficient.

Most RF systems are built on a 50 Ω impedance. Some systems use 75 Ω, which is more suitable for high-speed digital signals.

The quality of impedance matching can be mathematically expressed by the reflection coefficient (Gamma). A perfect match corresponds to Gamma=0, while a complete discontinuity (where all energy is reflected) corresponds to Gamma=1.

Another method to quantify impedance matching quality is standing wave ratio (VSWR).